The Untempered Chromatic Scale (Part 1)

The familiar 12-note scale is also called the chromatic scale. There are many ways to generate an untempered version of such a scale.

The oldest way seems to be Pythagorean tuning. When you multiply a frequency by 3, you get a new note, an octave plus a fifth above. Multiply by 3 again, and you get another new note, and so on. Dividing by 3 gives you another new note, the perfect fourth, dividing by 3 again delivers another new note, and so on. Do this enough times and you can generate a 12-tone scale.

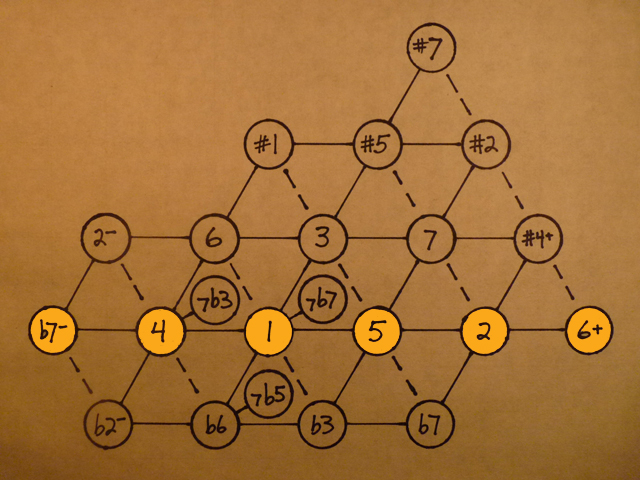
This scale forms the central, horizontal spine of the lattice.

 

The central six notes of the Pythagorean scale are highlighted. If you keep extending to the left and right you get all 12 notes, and then even more.

Trouble is, once you’re more than two or three steps away from the center, the notes are definitely dissonant. The ratios just get so big that the ear can no longer hear them as harmony. The Pythagorean major third, for example, is the next note to the right of the 6+, just off the central lattice. Its ratio is 3x3x3x3, or 81/1. The octave reduction trick allows you to divide by 2 until it’s in the same octave as the tonic, so the ratio becomes 81/64.

Harmony is built from small, whole number ratios, and these are not small numbers. Here is what a Pythagorean major third sounds like:

clarinet 81:64

Wow. Back when Pythagorean tuning was the norm, the third was considered a dissonant interval. I concur!

In late Medieval times, the 5/4 tuning became more popular, and major thirds began to be regarded as a consonant interval. Here’s the 5/4, for comparison:

clarinet 5:4

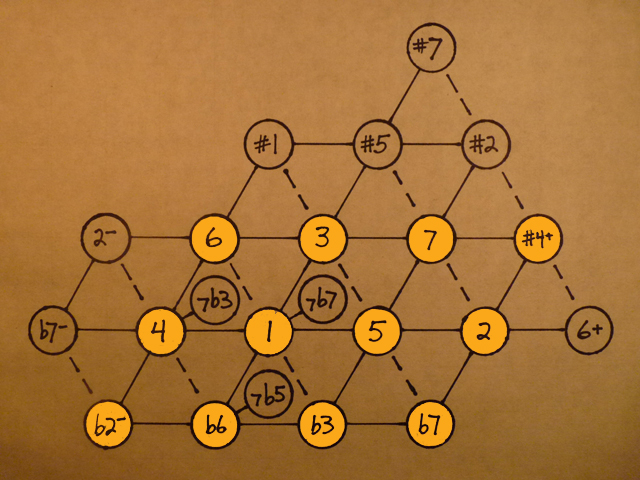
Ah. Using the prime number 5 allows a more consonant chromatic scale, with much smaller ratios. Here it is on the lattice:

 

All of these notes have been covered in previous posts. Each one has a unique personality. Some are more consonant, some more dissonant, but all twelve have small enough numbers in their ratios to be perceived as harmony by the ear.

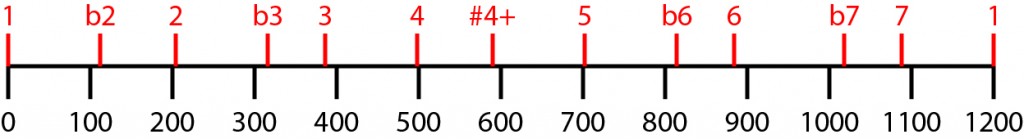
Here are their ratios, and pitches in cents:

1 — 1/1 — 0

b2 — 16/15 — 112

2 — 9/8 — 204

b3 — 6/5 — 316

3 — 5/4 — 386

4 — 4/3 — 498

#4+ — 45/32 — 590

5 — 3/2 — 702

b6 — 8/5 — 814

6 — 5/3 — 884

b7 — 9/5 — 1018

7 — 15/8 — 1088

Here is the scale, compared with the 12 notes of equal temperament.

 

When I first drew this scale, on graph paper, I was startled by how narrow the major-minor half steps are, compared with the equal-tempered versions. Take a look at the b3 (minor third) and the 3 (major third). They are only 70 cents apart, not the 100 cents of the piano scale. Same with the b6-6 and b7-7. It’s such a small move in pitch, such a large move harmonically!

The Mozart movies I made a month ago or so illustrate the major-minor pair quite well. The blandification of equal temperament has obscured a beautiful detail of harmonic music. So much is gained with ET, and so much is lost or obfuscated.

Next: The Untempered Chromatic Scale (Part 2)