The Major Scale in Cents
The simplest untempered tuning of the major scale is:
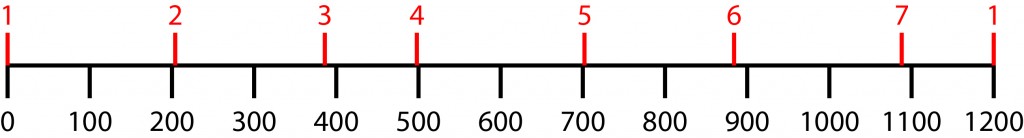
1 — 0 cents
2 — 204
3 — 386
4 — 498
5 — 702
6 — 884
7 — 1088
Here’s how that tuning compares with the equal tempered scale:
The black numbers show the pitches of 12-tone equal temperament. They are equally spaced, like inches on a ruler.
The red numbers show the tuning of the untempered major scale. They are spaced in the way they naturally turn out when you generate them with small whole number ratios. As is so often the case with the natural world, they don’t line up too well with the nice human grid lines we love.
The way I see it, when you play in equal temperament, you’re playing the grid lines on the map.
When you sing or play the untempered notes, you are visiting the actual territory.
I’ve read that it’s not possible to combine the two, but I disagree. It’s a matter of showing the ET instrument who’s the boss. My favorite example is Ray Charles. Here’s a video from 1976. He’s playing the piano, laying down those grid lines, and the rest of the band is too, but when he sings, his voice owns the sound, and the sound becomes him. A great, dominant singer will infuse the whole combo with that soul.
Another great example is Ella Fitzgerald. Want some goosebumps? Check this video out.
I was studying this with a view to tuning a diatonic harmonica. My tuner app which tells how many cents a note is sharp or flat compared to equal temperament. In equal temperament tuning the chords on the lower end of the harmonica sound harsh. I need to work out exactly how many cents each harmonic note is from its tempered equivalent.
Interesting inquiry, never thought of that. The harmonica notes that are audibly off in ET are the 3, 6 and 7. All should be tuned a little flat (14, 16 and 12 cents respectively) and in straight harp the three main chords should sound great. Don’t know about other chords, or cross harp, you might get a wolf note or two. Happy experimenting!